resistors in parallel equation When dealing with electrical circuits, one essential concept is the “resistors in parallel equation.” This equation is vital for calculating the total resistance while multiple resistors are linked in parallel.
Whether you are a scholar, hobbyist, or professional in electronics, knowledge of this equation lets you design and troubleshoot circuits greater effectively. In this complete guide, we can explore the resistors in parallel equations in detail, imparting clean reasons and sensible examples.
key takeaway
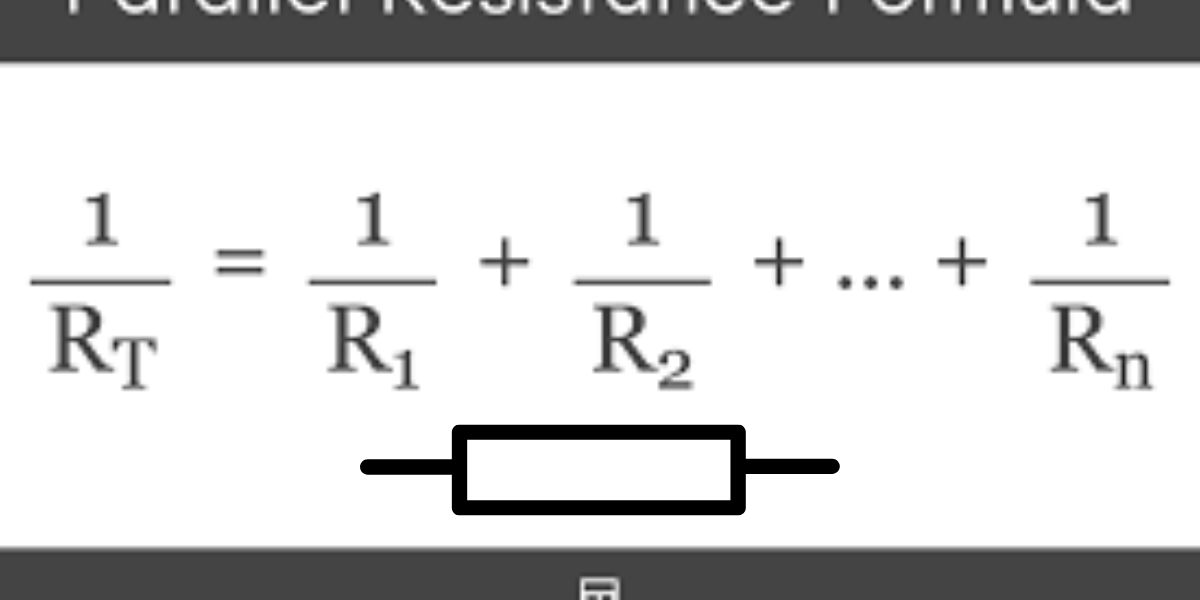
Mathematically, if R1,R2,R3,…,RnR_1, R_2, R_3, \ldots, R_n are resistors connected in parallel, the equivalent resistance ReqR_{\text{eq}} is given by:
1Req=1R1+1R2+1R3+⋯+1Rn\frac{1}{R_{\text{eq}}} = \frac{1}{R_1} + \frac{1}{R_2} + \frac{1}{R_3} + \cdots + \frac{1}{R_n}
To find ReqR_{\text{eq}}, calculate:
Req=1(1R1+1R2+1R3+⋯+1Rn)R_{\text{eq}} = \frac{1}{\left(\frac{1}{R_1} + \frac{1}{R_2} + \frac{1}{R_3} + \cdots + \frac{1}{R_n}\right)}
This formula indicates that:
- The total resistance in a parallel circuit is always less than the smallest resistance among the parallel resistors.
- Adding more resistors in parallel decreases the total resistance.
This is crucial for understanding how resistors affect each other in a circuit and for designing circuits with specific resistance values.
What are the Resistors in Parallel Equation?
What are the Resistors in Parallel Equation?
The resistors in parallel conditions are utilized to choose the identical resistance of resistors connected in a parallel setup. When resistors are related in parallel, the full resistance isn’t truly the sum of the person’s resistances. Instead, the reciprocal of the entire resistance is equal to the sum of the reciprocals of each character resistor’s resistance.
The Formula for Resistor in Parallel
To find the equivalent resistance ReqR_{eq} of resistors in parallel, you use the following formula:
1Req=1R1+1R2+1R3+⋯+1Rn\frac{1}{R_{eq}} = \frac{1}{R_1} + \frac{1}{R_2} + \frac{1}{R_3} + \cdots + \frac{1}{R_n}
Where:
- ReqR_{eq} is the equivalent resistance of the parallel resistors.
- R1, R2, R3,…, RnR_1, R_2, R_3, \ldots, R_n are the resistances of the individual resistors.
After calculating the sum of the reciprocals, you take the reciprocal of the result to find ReqR_{eq}.
Example Calculation
Suppose you have 3 resistors linked in parallel with resistances of four ohms, 6 ohms, and 12 ohms. To locate the equal resistance, you follow the resistors in the parallel equation as follows:
Calculate the reciprocal of each resistor’s resistance:
- 1R1=14=0.25\frac{1}{R_1} = \frac{1}{4} = 0.25 1R2=16≈0.167\frac{1}{R_2} = \frac{1}{6} \approx 0.167 1R3=112≈0.083\frac{1}{R_3} = \frac{1}{12} \approx 0.083
- Add these values together:1Req=0.25+0.167+0.083=0.5\frac{1}{R_{eq}} = 0.25 + 0.167 + 0.083 = 0.5
- Take the reciprocal of the result:Req=10.5=2 ohmsR_{eq} = \frac{1}{0.5} = 2 \text{ ohms}
Thus, the equivalent resistance of the three resistors in parallel is 2 ohms.
Why Use the Resistors in Parallel Equation?
Understanding the resistors in parallel equation is essential for several motives:
Reduced Total Resistance
In parallel circuits, the entire resistance is constantly much less than the smallest resistor in the institution. This is beneficial when you need to decrease the general resistance in a circuit without changing existing resistors.
Increased Current Handling
By connecting resistors in parallel, you successfully grow the circuit’s capability to handle greater contemporary. This is especially useful in energy distribution systems wherein excessive present-day flow is essential.
Voltage Division
Parallel resistors share the same voltage throughout them, which allows for specific voltage drops to be carried out in line with the design necessities.
Applications of Resistors in Parallel
Applications of Resistors in Parallel
The resistors in parallel equations are extensively implemented in diverse fields:
Power Supplies
In energy deliver circuits, resistors are regularly linked in parallel to reap the favored resistance and energy coping capabilities.
Electronics Design
Circuit designers use parallel resistors to high-quality-track resistance values, regulate modern-day flow, and manipulate voltage drops.
Load Balancing
In electric engineering, resistors in parallel are used to balance masses across a couple of paths to ensure the efficient distribution of electrical electricity.
Troubleshooting Common Issues
When running with resistors in parallel, you may come across some commonplace problems:
Incorrect Calculations
Ensure you effectively practice the resistors in parallel equations and double-take a look at your math to avoid calculation mistakes.
Component Tolerances
Resistors have tolerances that can affect their actual resistance values. Be aware of these tolerances whilst calculating the equal resistance.
Overheating
Improper resistor rankings or excessive modern can cause overheating. Make certain resistors are rated accurately for the modern-day they will bring.
FAQs
What is the Contrast Between Resistors in Arrangement and Parallel?
In a chain circuit, resistors are associated end-to-give-up, and the full resistance is the entirety of the man or lady resistances. In a parallel circuit, resistors are associated all through the indistinguishable two focuses, and the general resistance is found utilizing the resistors in a parallel equation.
Can You Utilize the Resistors in Parallel Conditions for Any Number of Resistors?
Yes, the resistors in parallel conditions may be utilized for any amount of resistors. You without a question need to highlight the reciprocals of all of the resistances after which take the complementary of the result.
What Happens if One Resistor in a Parallel Circuit Comes up short?
If one resistor fails to open in a parallel circuit, the overall resistance will increase, however, the circuit will still be characteristic, as the ultimate resistors retain to offer a path for cutting-edge.
How Does Temperature Affect Resistors in Parallel?
Temperature changes can affect resistor values. As temperature increases, resistors can change resistance slightly due to temperature coefficients. This can affect the accuracy of your calculations.
Conclusion
The resistors in parallel equations are an essential concept in electrical and digital engineering. It permits the accurate calculation of overall resistance whilst resistors are related in parallel. By know-how and applying this equation, you could design circuits that meet your unique needs for resistance, current managing, and voltage division.
Remember to use the formulation efficaciously, keep in mind the impact of tolerances and temperature adjustments, and make certain your calculations are accurate. Whether you’re constructing simple circuits or complicated systems, getting to know the resistors in parallel equations will beautify your ability to paint correctly with electrical components.
