Consider a Charged Parallel-Plate Capacitor, When managing with a charged parallel-plate capacitor, understanding how to alter its capacitance is basic for different applications in gadgets and material science.
In this comprehensive article, we’ll investigate how to split the capacitance of a parallel-plate capacitor, which is a pivotal concept for anybody working with electrical circuits.
What is a Parallel-Plate Capacitor?
What is a Parallel-Plate Capacitor?
Before diving into methods to halve its capacitance, let’s briefly review what a parallel-plate capacitor is. A parallel-plate capacitor consists of two conductive plates separated by an insulating material, known as a dielectric. The capacitance CC of a parallel-plate capacitor is determined by the formula:
C=ε0⋅εr⋅AdC = \frac{{\varepsilon_0 \cdot \varepsilon_r \cdot A}}{d}
where:
- ε0\varepsilon_0 is the permittivity of free space,
- εr\varepsilon_r is the relative permittivity of the dielectric material,
- AA is the area of the plates,
- dd is the distance between the plates.
Why Divide the Capacitance?
There are a few reasons why you might need to split the capacitance of a parallel-plate capacitor. In a circuit plan, capacitance needs to be balanced to meet particular prerequisites, such as tuning thunderous circuits or optimizing channel reactions. Dividing the capacitance can offer assistance in fine-tuning circuits to accomplish desired electrical characteristics.
How does the capacitance of the parallel plate capacitor change if the separation between the plates is halved?
When the separation between the plates of a parallel-plate capacitor is halved, the capacitance of the capacitor increases.
This relationship can be understood through the fundamental capacitance formula for a parallel-plate capacitor, which is given by C=ε0⋅εr⋅AdC = \frac{\varepsilon_0 \cdot \varepsilon_r \cdot A}{d}.
In this formula, ε0\varepsilon_0 represents the permittivity of free space, εr\varepsilon_r is the relative permittivity of the dielectric material between the plates, AA is the area of the plates, and dd is the separation between them.
According to this formula, capacitance CC is inversely proportional to the distance dd between the plates. This means that if the distance dd is reduced, the capacitance CC increases proportionally.
Specifically, if the separation between the plates is halved (i.e., the new distance is d2\frac{d}{2}), the capacitance becomes:
C′=ε0⋅εr⋅Ad2=2⋅ε0⋅εr⋅Ad=2CC’ = \frac{\varepsilon_0 \cdot \varepsilon_r \cdot A}{\frac{d}{2}} = 2 \cdot \frac{\varepsilon_0 \cdot \varepsilon_r \cdot A}{d} = 2C
Thus, halving the separation between the plates results in doubling the capacitance of the parallel-plate capacitor.
This principle is crucial for adjusting capacitors in various electronic applications, where changing the plate separation is one of the methods to fine-tune the capacitance to desired levels.
How to Divide the Capacitance of a Parallel-Plate Capacitor
How to Divide the Capacitance of a Parallel-Plate Capacitor
To accomplish the objective of dividing the capacitance in a parallel-plate capacitor, you can utilize a few approaches. Here are the most compelling methods:
1. Diminish the Plate Area
The capacitance of a parallel-plate capacitor is straightforwardly corresponding to the zone of the plates. Hence, to split the capacitance, you can decrease the plate range by half.
If the unique plate zone is ( A ), at that point diminishing it to ( frac{A}{2} ) will result in a modern capacitance ( C’ ) given by:
C′=dε0⋅εr⋅2A=2C
This strategy is viable but may not continuously be doable depending on the plan imperatives of the capacitor or circuit.
2. Increment the Remove Between Plates
Another way to split the capacitance is by expanding the separation between the plates. The capacitance is contrarily relative to the separate ( d ) between the plates. If you increment ( d ) to ( 2d ), the unused capacitance ( C’ ) will be:
C′=2dε0⋅εr⋅A=2C
This strategy is frequently utilized in flexible capacitors or tuning applications where changing the separation between plates is practical.
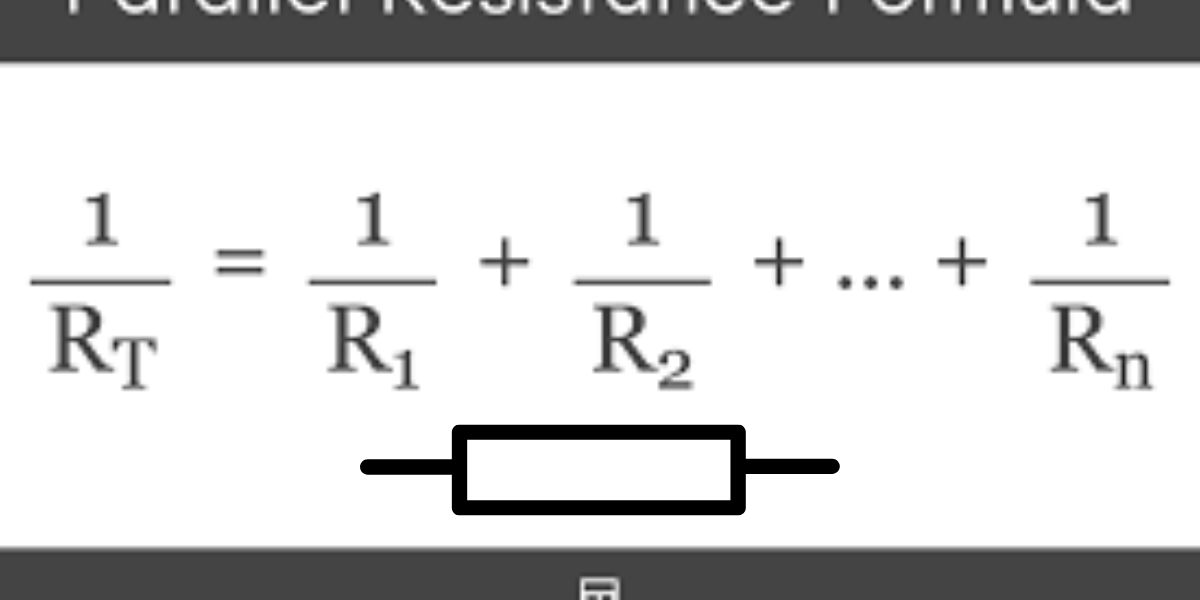
| Method | Description | Formula Impact |
|---|---|---|
| 1. Decrease the Plate Area | Reduce the area of the capacitor plates while keeping the distance between them constant. | C∝AC \propto A (Capacitance is directly proportional to plate area) |
| 2. Increase the Plate Separation | Increase the distance between the capacitor plates while keeping the plate area constant. | C∝1dC \propto \frac{1}{d} (Capacitance is inversely proportional to the separation distance) |
| 3. Reduce the Dielectric Constant | Use a dielectric material with a lower dielectric constant, or remove the dielectric material if present. | C∝1κC \propto \frac{1}{\kappa} (Capacitance is inversely proportional to the dielectric constant) |
3. Supplant the Dielectric with a Fabric of Lower Relative Permittivity
The capacitance of a parallel-plate capacitor is moreover corresponding to the relative permittivity ( varepsilon_r ) of the dielectric fabric.
By supplanting the dielectric fabric with one that has half the relative permittivity of the unique fabric, you can accomplish the craved dividing of the capacitance.
For occasion, if the unique dielectric fabric has ( varepsilon_r ), exchanging to a dielectric with ( frac{varepsilon_r}{2} ) will result in:
C′=dε0⋅2εr⋅A=2C
This strategy is valuable in circumstances where changing the dielectric fabric is practical.
4. Utilize a Arrangement Combination of Capacitors
If you’re managing with numerous capacitors, you can accomplish a general capacitance that is half of a single capacitor by interfacing capacitors in the arrangement.
For case, if you have two indistinguishable capacitors each with capacitance ( C ), interfacing them in the arrangement will result in an add-up to capacitance ( C_{total} ) of:
Ctotal1=C1+C1=C2
So, the add-up to capacitance ( C_{total} ) will be:
Ctotal=2C
This approach is frequently utilized in viable electronic circuits to alter by and large capacitance.
How can you reduce the capacity of parallel capacitor separation between two plates?
How can you reduce the capacity of parallel capacitor separation between two plates?
To reduce the capacitance of a parallel-plate capacitor by increasing the separation between the two plates, you need to understand the relationship between capacitance and plate separation.
The capacitance \( C \) of a parallel-plate capacitor is given by the formula:
\[ C = \frac{\varepsilon_0 \kappa A}{d} \]
where \( \varepsilon_0 \) is the permittivity of free space, \( \kappa \) is the dielectric constant of the material between the plates, \( A \) is the area of the plates, and \( d \) is the separation distance between the plates.
According to this formula, capacitance is inversely proportional to the separation distance \( d \). This means that if you increase the distance \( d \) between the plates, the capacitance \( C \) will decrease. Specifically, doubling the separation distance \( d \) will result in the capacitance being halved.
To achieve this in practice, you would physically adjust the setup of the capacitor to increase the gap between the plates.
This can be done by either extending the supports that hold the plates apart or by adding insulating spacers to increase the distance between the plates.
As you increase the separation, the capacitance decreases in a manner inversely proportional to the increase in distance.
Down to earth Considerations
When endeavoring to divide the capacitance of a parallel-plate capacitor, consider the after viable factors:
- Space and Plan Limitations: Diminishing plate range or expanding plate partition might not continuously be doable due to physical space imperatives or plan limitations.
- Fabric Accessibility: Supplanting dielectrics requires accessibility of reasonable materials with the craved permittivity values.
- Exactness: Alterations to capacitor values ought to be exact, particularly in high-frequency applications, to guarantee precise performance.
FAQs
Can I split the capacitance of a parallel-plate capacitor by essentially splitting the plate area?
Yes, lessening the plate range to half will specifically divide the capacitance of the parallel-plate capacitor.
Is it conceivable to split the capacitance by expanding the remove between the plates?
Absolutely. Multiplying the remove between the plates will divide the capacitance.
How does changing the dielectric fabric influence capacitance?
Replacing the dielectric fabric with one of lower relative permittivity will diminish the capacitance. Dividing the permittivity of the dielectric will result in splitting the capacitance.
Can capacitors be combined to accomplish half the capacitance?
Yes, interfacing capacitors in the arrangement will accomplish an add-to capacitance that is less than each person’s capacitor.
Two indistinguishable capacitors in arrangement will result in an added capacitance of half the esteem of one capacitor.
Conclusion
In outline, if you require to divide the capacitance of a charged parallel-plate capacitor, there are a few successful strategies to accomplish this objective. You can diminish the plate zone, increment the removal between the plates, supplant the dielectric with a fabric of lower relative permittivity, or utilize an arrangement combination of capacitors.
Each strategy has its possess commonsense contemplations and can be chosen based on the particular prerequisites of your application.
Understanding these strategies not as it were makes a difference in the exact circuit plan but moreover upgrades your general handle on capacitor usefulness in electronic frameworks.
Whether you’re planning channels, tuning circuits, or testing with electrical components, knowing how to alter capacitance effectively is an important aptitude in gadgets.